Category: Mathematics

Further iterations

Xaos

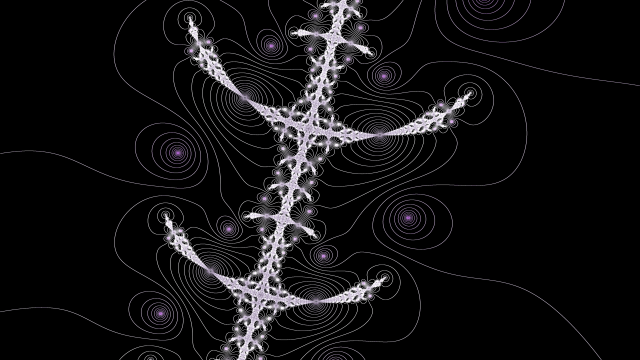
I recently checked to see if there are any new fractal generators since I last played with them. Apparently not, at least for Macs not running the latest OS. Ultra Fractal is still the most capable. It’s pricey, but it does give you a month’s free trial. Xaos works well also, and it’s free.

Fractal Domains and Fraqtive have not been updated since I last used them over five years ago. It’s possible to make pretty, complicated pictures with them, but it’s a struggle, and to my eyes the results from the other two applications look better. Both are free now, so check them out if you’re curious.

Ultra Fractal
Fractal Domains
Fraqtive

For all of these, right-click and open in a new window to see every little detail.

Continue reading “Further iterations”

W. Heath Robinson expects an apology

Spare room

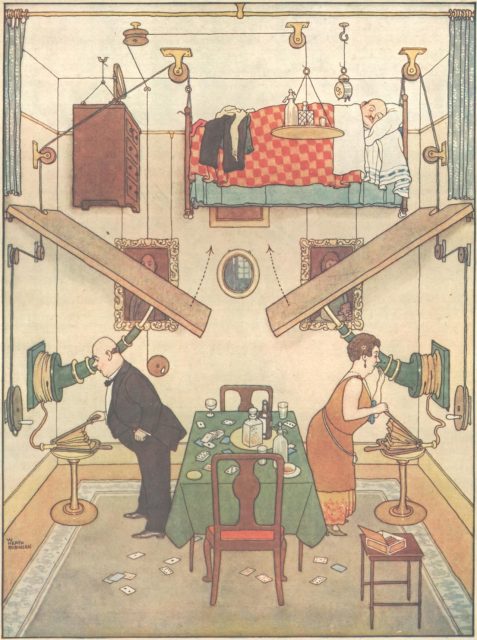
In a Heath Robinson device, everything has a clear purpose, no matter how strange it appears. For example, there is a clearly discernable logic to the profusion of cables, pulleys and bellows in his “Spare Room,” above, from this year’s HR calendar. It may not be realistic — I wouldn’t want to sleep in that bed — but everything makes sense. (The same is true of the inventions of Heath Robinson’s American counterpart, Rube Goldberg.) Comparing Neil Ferguson’s incomprehensible mathematical model to a Heath Robinson device slanders Robinson.

Bonus calendar picture: Uncle Lubin in color.

Uncle Lubin

Suggestion

Stuck at home because of the CCP virus? Why not follow Isaac Newton’s example and use the time to revolutionize a field of study or two:

Soon after Newton had obtained his BA degree in August 1665, the university temporarily closed as a precaution against the Great Plague. Although he had been undistinguished as a Cambridge student, Newton’s private studies at his home in Woolsthorpe over the subsequent two years saw the development of his theories on calculus, optics, and the law of gravitation.

Notes on the perception of imaginary differences

Here’s a MIDI file of Scarlatti’s Sonata in A Major, K24/L495/P80, played on a physically-modeled virtual harpsichord tuned to A = 440 Hz:

Here it is again, this time with A = 432 Hz:

Did the first recording make you feel “self-centered, narcissistic, materialistic and aggressive“? Did the second resonate with the Heart Chakra, repair your DNA and restore your spiritual and mental health? If so, I congratulate you on your acute sensitivity. (Be sure to wear protective headgear at all times.)

Or did the second just sound a little flatter than the first?

Even if there is a real basis to the paranoid theories — extremely unlikely; the rise of the 440 standard is so complicated that positing a vast international conspiracy is inadequate to explain it — the precise frequency of the “A” in a scale matters far less than the qualities of the intervals between the notes of the scale.

The preset used for the two recordings above does not specify the temperament, which implies that it is equal-tempered. Other presets offer different tuning systems. Here is the sonata again, this time at A = 415, using an unspecified “well tempered” tuning:

And again, at A = 392, using “Werckmeister III“:

Even ordinary human beings who don’t wear tin-foil hats might be able to hear subtle differences in the character of the music now.

(Via Dustbury.)

Gotta have a Feckle Freezer

Accumulated odds and ends:

Is Obama Catholic? No, and Dennis McDonough is an idiot.

Is the Pope Catholic? That’s a much more interesting question. Edward Feser supplies some useful background, including notes about Popes Honorius, John XXII and Liberius.

Hyperplay will provide hours — well, minutes — of fun for the mathematically inclined and the easily entertained.

Continue reading “Gotta have a Feckle Freezer”

Evil and odious

Years ago, when I was a youngster in Bigamy City, Utah1, I played games stepping on the cracks between sidewalk blocks while walking home from the park. My favorite was as follows:

Step on a crack with the right foot.

Step on a crack with the left foot.

Reverse that: left, then right.

Take the entire sequence so far and reverse it: left, right, right, left.

Now take the entire eight-step sequence, and reverse that: left, right, right, left, right, left, left, right.

And so on.

I could usually keep the sequence straight for 64 cracks or so, but eventually I would either be distracted by something or run out of sidewalk. Later I found that if you arranged black and white boxes in a square grid using the sequence, the sides of which square were a power of two, you’d get a pattern with a high degree of symmetry. You could also use “0” and “1” to represent the elements of the sequence, which led to other sorts of games.

Morse-Thue_sequence

Last night, while browsing around online, I discovered that my game does in fact have a name: the Thue-Morse sequence. I was amused to see that one of the ways of generating it involves the notion of “evil” and “odious” numbers.

Recursive excursions

Fractal domains

I recently checked out some more fractal generators for Macs. The most capable of this batch was Fractal Domains, which has numerous options for stylizing and coloring the fractal. It costs $20 if you want to export the results, though you can grab screen captures if you’re really cheap. The creator’s to-do list includes adding the capability of recording animations, but that is probably two updates away.

Fractal Domains
Fractal Domains

Fraqtive only does Mandelbrot and Julia sets, and it has fewer options for coloring. However, it’s free. The most recent version is capable of recording animations, but that version hasn’t been ported to the Mac yet.

Fraqtive
Fraqtive

The images Fractal Architect generates look more like abstract expressionism than mathematics. There is a free version, but it has severe limits on exporting. The interface is confusing, and trial-and-error doesn’t quickly yield interesting results.

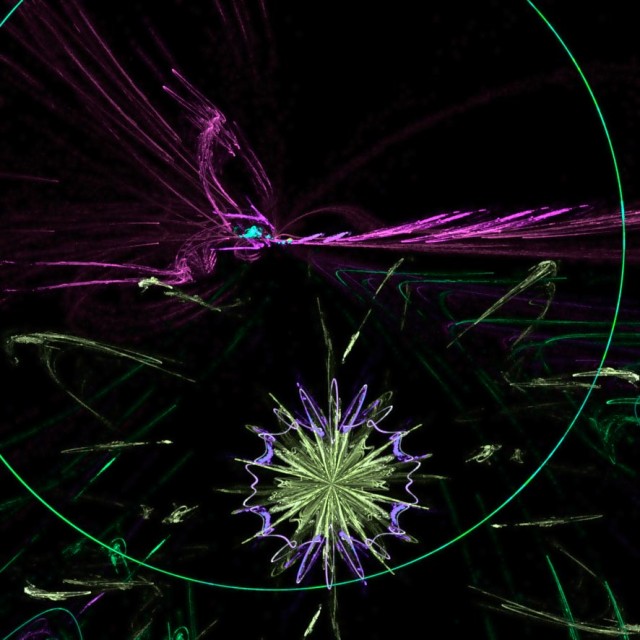
Fractal Architect
Fractal Architect

Fractal Domains and Fraqtive are worth trying if you are interested in psychedelic paisley and endlessly intricate designs. However, the generators I looked at previously are probably better choices, and both of them, Xaos and Ultra Fractal, are capable of recording animations.

Continue reading “Recursive excursions”

Zooming along

Back in the 1980s, it took immense amounts of computer time to generate fractal images. If you wanted to see pretty pictures of recursively-generated figures, you borrowed books such as The Beauty of Fractals, or watched Nothing But Zooms if you knew someone with the video. Computers and software have come a long way since then, and nowadays you can easily create your own fractal images on your laptop. I experimented with a couple of applications for Macs this weekend, Xaos and UltraFractal.

Xaos is freeware, and it comes in Windows and Linux versions as well. It’s fairly easy to use, though you might want to bookmark the online documentation. It’s what I used to make the video above. It doesn’t actually make movies. Instead, it outputs a series of .png files, which you can import into Final Cut or something similar. (Be aware that at 30 frames per second, there are 1800 images per minute. You’re going to be working with a lot of files.)

Ultra Fractal has some Photoshop-style image manipulation features. It’s more complicated than Xaos but not really difficult to figure out. However, it’s a bit pricey; if you want to do animations, it will cost you $130. I think I’ll make do with Xaos for now.

Julia set in Ultra Fractal